Evidence of Understanding
- describe proportional relationships between angles, arcs, and sectors of a circle
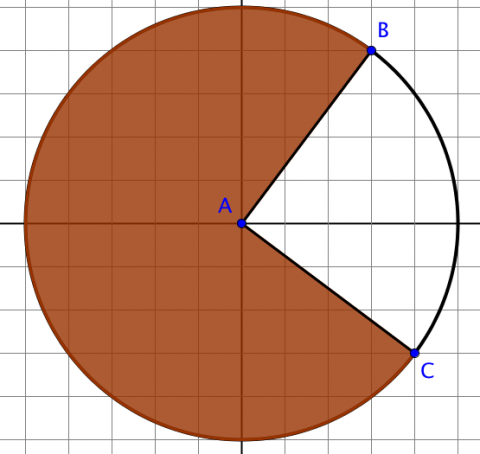
- create visuals to describe a central angle in terms of the fraction of the circle it represents
- Example: show an angle measuring 72° is equivalent to ⅕ of the circle
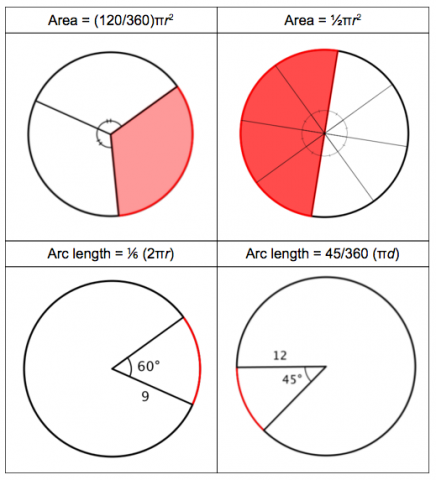
- investigate the constant ratio between an angle’s arc length and a circle’s circumference
- explain the proportional relationship with the radius
- use similarity to derive the length of the arc intercepted by an angle
- use tools to verify the relationship between an angle’s degree and radian measure
- apply the relationship between degrees and radians to find a missing measure
- determine and justify the length of 1 radian
- explain how sectors are related to area of a circle using similarity relationships
- derive and use the formula for determining the area of a sector
- derive and use the formula for determining the area of a sector
- create visuals to describe a central angle in terms of the fraction of the circle it represents
- analyze and apply the relationships between the angles and arcs used to describe a circle
- use examples and nonexamples to distinguish central, inscribed, and circumscribed angles
- define and classify angles using radii, chords, and tangents
- investigate relationships between angle and arc measure for a central, inscribed, or circumscribed angle and use them to solve problems
- prove inscribed angles open to the same arc are congruent (and vice versa)
- prove parallel chordsintercept congruent arcs
- prove that a triangle inscribed in a circle with a leg through the center of the circle is a right triangle (hypotenuse is the diameter)
- describe patterns relating the angle and arc measures on a circle by two tangents, two secants, a secant and a tangent, or a chord and a tangent
- describe patterns relating the angle and arc measures resulting from two intersecting chords
- calculate the value of an unknown angle or arc measure
- use examples and nonexamples to distinguish central, inscribed, and circumscribed angles
Develop conceptual understanding:
central angle, arc length, circumference, degrees, radians, sector, inscribed angle, circumscribed angle
Supporting terms to communicate:
equivalent, ratio, proportion, similarity, scale factor, dilation, angle, radius, diameter, area, chord, tangent, secant, parallel, alternate interior angles, hypotenuse