Families of Functions

11 Components
Initial Task
Sorting Functions
Big Ideas
Big Idea 1 Big Idea 2 Big Idea 3 Big Idea 4 Big Idea 5
Formative Assessment Lesson
Functions & Situations
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 1 Performance Task for Unit 1 End of Unit Assessment Teacher Resources ALG II Unit 1 Find aligned Regents questions with Quiz Banker
A2 U1: Families of Functions
Browse Components
Initial Task
Sorting Functions
Big Ideas
Big Idea 1 Big Idea 2 Big Idea 3 Big Idea 4 Big Idea 5
Formative Assessment Lesson
Functions & Situations
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 1 Performance Task for Unit 1 End of Unit Assessment Teacher Resources ALG II Unit 1 Find aligned Regents questions with Quiz Banker
Big Idea:

Big Idea 4

An inverse function is a function that "undoes" another function; if f(x) maps x to y, then its inverse maps y back to x.

1 week
See Standards
Common Core: Major Standards:
F-IF.B.4

Interpret functions that arise in applications in terms of the context. For a function that models a relationship between two quantities, interpret key features of graphs and tables in terms of the quantities, and sketch graphs showing key features given a verbal description of the relationship. Key features include: intercepts; intervals where the function is increasing, decreasing, positive, or negative; relative maximums and minimums; symmetries; end behavior; and periodicity.

Common Core: Additional Standards:
F-BF.B.3

Build new functions from existing functions Identify the effect on the graph of replacing f(x) by f(x) + k, kf(x), f(kx), and f(x + k) for specific values of k (both positive and negative); find the value of k given the graphs. Experiment with cases and illustrate an explanation of the effects on the graph using technology. Include recognizing even and odd functions from their graphs and algebraic expressions for them.

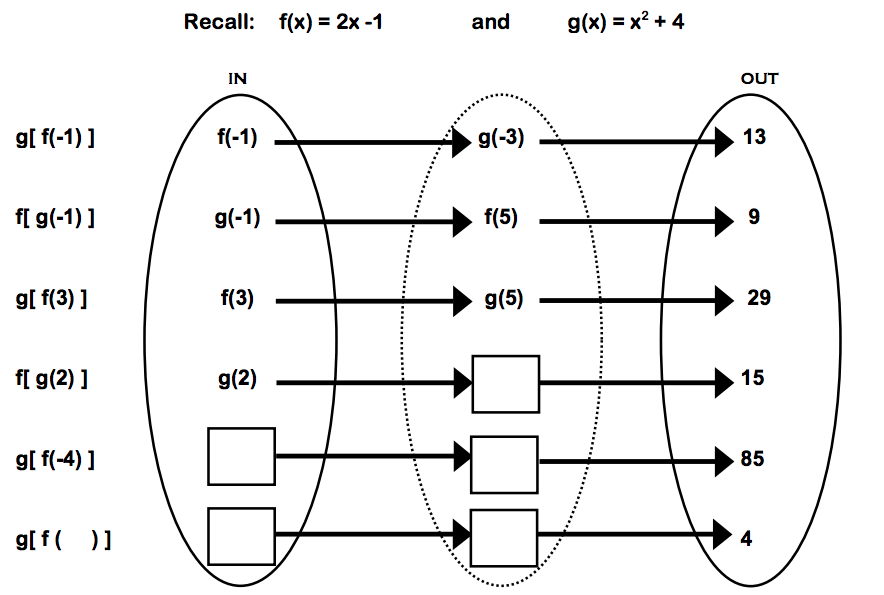
Evidence of Understanding

  • use composition of functions to prove that two functions are inverses
    • justify why the output of the composition of inverse functions is the input
    • describe how operations on the function's domain are counterbalanced in its inverse function
      • explain why quadratic and square root functions are inverses
      • Example: y = 2x2 - 5 has an inverse with +5,●½, and a square root
    • <explain>only inverse functions have commutative compositions</explain>
  • create a function’s inverse using a graph or table of values
    • justify two functions are inverses using specific points from the tables of each function
    • describe the general shape and characteristics of a given function's inverse
      • use multiple representations of a function to help illuminate characteristics of its inverse
    • given a function's graph, create a graph or table of values for its inverse function
    • determine whether a function is one to one and has an inverse function
      • explain when domain needs to be restricted to produce an inverse and state the restriction (radical functions and the difference between square root and cube root)
    • explain why inverse functions reflect over the line y = x
  • generate an equation for the inverse of a function and use it to solve problems
    • confirm two equations are inverses using compositions of functions or the reflection over y = x
    • given a function's graph or table of values, create and justify the function's inverse equation
      • use rate of change and other key characteristics to create the inverse equation
    • algebraically generate the inverse equation (Note: only for simple polynomials- especially linear, quadratic and cubic, or their inverses- radical, cube root, etc.)
    • use the inverse equation to describe qualities about the graph or table of f(x) or f-1(x)
      • solve square root equations and explain extraneous solutions

Develop conceptual understanding:

inverse function, commutative, one to one, radical function, square root, cube root, reflect, extraneous solution

Supporting terms to communicate:

composition, input, output, independent, dependent, domain, range, symmetry, linear, quadratic, cubic

Core Resource
A core resource supports multiple days of instruction.
Instructional Routine: Connecting Representations
These tasks are embedded within the instructional routine called Connecting Representations.