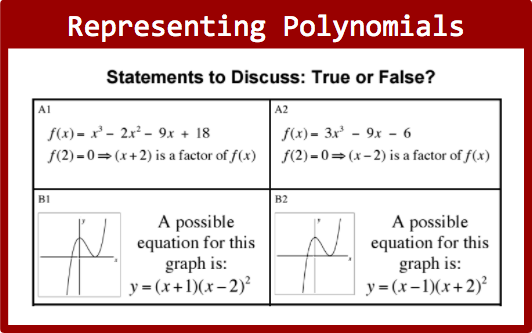
In this unit, students will study properties of rational and polynomial expressions as they appear in both functions and equations. They will also use solving polynomial equations of various forms to extend the number system into the Complex Plane. Students will identify algebraic terms, write equivalent expressions in factored form, and use the zero product property to understand that all polynomials can be expressed as a product of factors added to a remainder. This allows us to arrive at the remainder theorem, to identify the zeroes of the polynomial, and to sketch its graph.
Essential Questions:
- How do changes in the symbolic representation affect the graphical representation of the function?
- What are the key features of a polynomial function and what do they mean in the context of a problem?
- How do the properties of real numbers apply to imaginary numbers?
- How can polynomial, radical, or rational, functions model problems?
- Why are solutions considered “extraneous?"
- How do rational and polynomial functions occur?