Evidence of Understanding
- describe the characteristics of any circle
- construct a circle using a compass and justify that all radii are equidistant and congruent
- explain that all points on the circle are equidistant from the center of the circle
- define radius as a relationship between a circle’s center and points on the circle
- justify circles are similar using scale factor
- reason proportionally and connect the measurements of a circle with similarity
- analyze examples and non-examples to distinguish radii, chords, tangents, and secants
- use constructions to explain the relationship between the radius and a tangent line
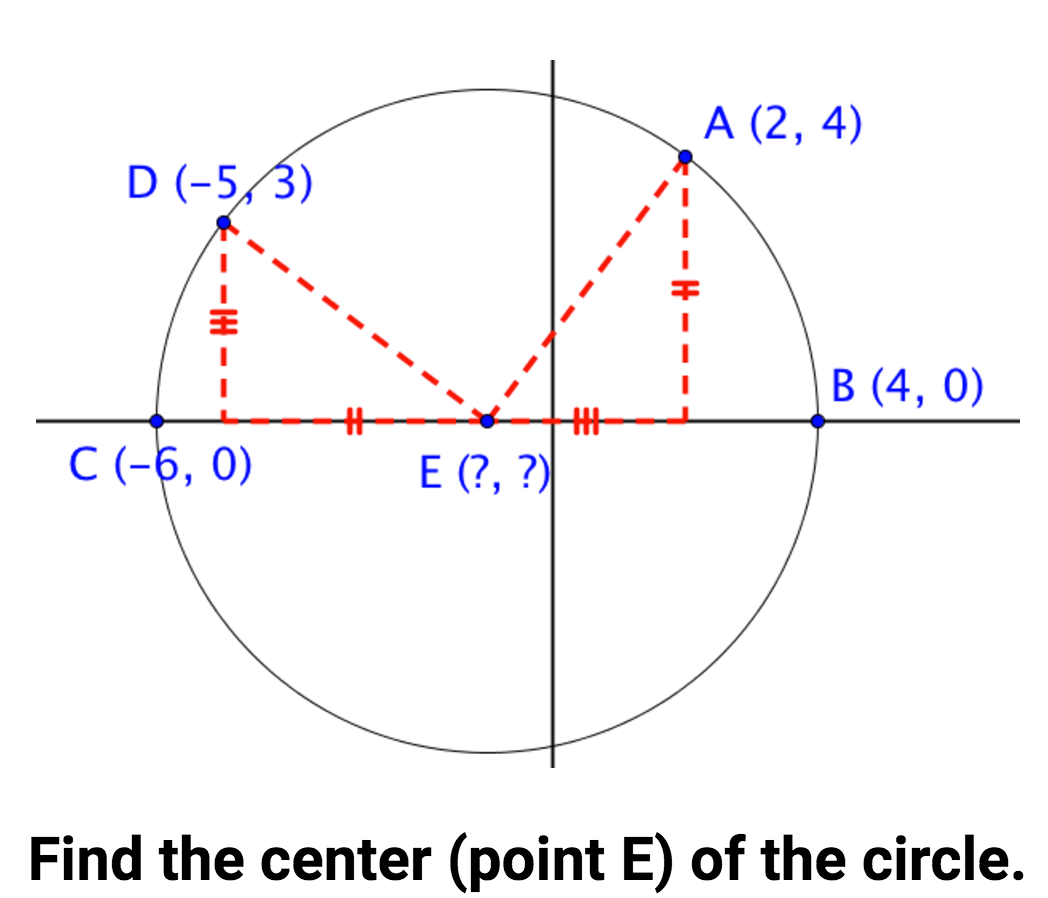
- construct perpendicular bisectors of chords to show they always pass through the circle’s center
- analyze characteristics of a circle in the coordinate plane
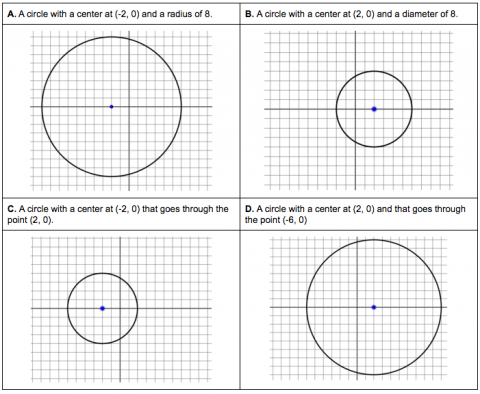
- graph a circle in the coordinate plane given its center and radius
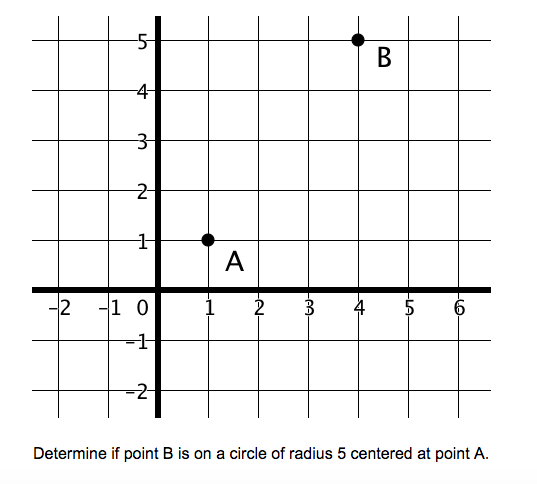
- explore whether a point lies on a circle whose radius and center point are known
- identify and justify possible methods including the use of a compass, straightedge, the Pythagorean Theorem, the distance formula, etc.
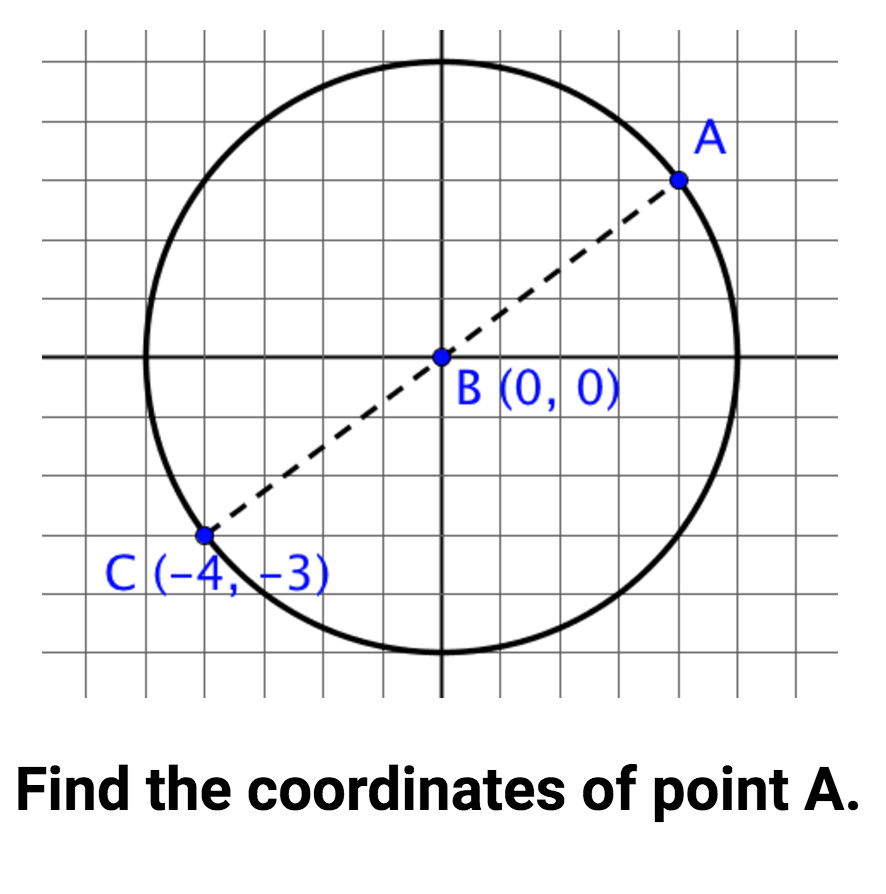
- prove whether a point lies on a circle given the circle’s center and another point on the circle
- describe characteristics about a circle given its center, radius, points on the circle, etc.
- Example: find the area of a circle whose endpoints of its diameter lie at (2, 3) and (10, -3), or determine the diameter of a circle centered at the origin and passing through (-5, 10)
- Example: find the area of a circle whose endpoints of its diameter lie at (2, 3) and (10, -3), or determine the diameter of a circle centered at the origin and passing through (-5, 10)
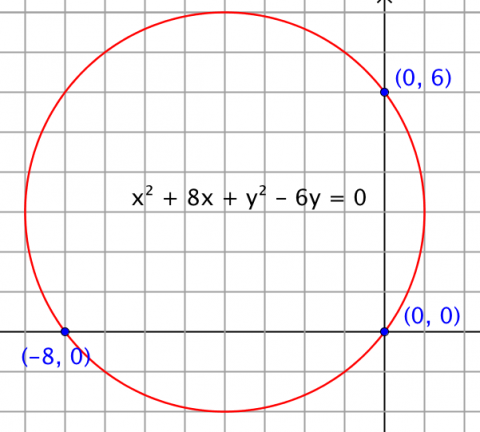
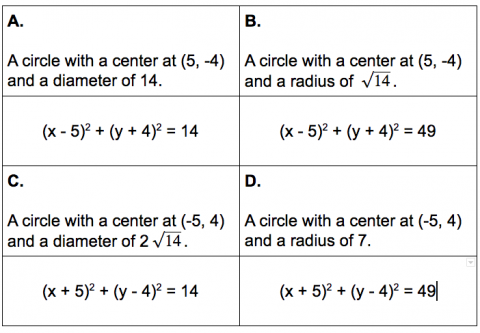
- determine the standard equation of a circle in the coordinate plane
- describe connections between a circle’s equation and and its graph in the coordinate plane
- generate the equation of a circle from its graph
- derive the equation of a circle from the Pythagorean Theorem
- describe the relationship between x, h, y, k,r and the Pythagorean Theorem
- generate a circle’s equation given information about its center, radius, or points on the circle
- apply completing the square to write the equation of a circle in standard form
Develop conceptual understanding:
circle, radius/radii, equidistant, center of the circle, points on the circle, equation of a circle
Supporting terms to communicate:
similar, scale factor, coordinates, Pythagorean Theorem, completing the square