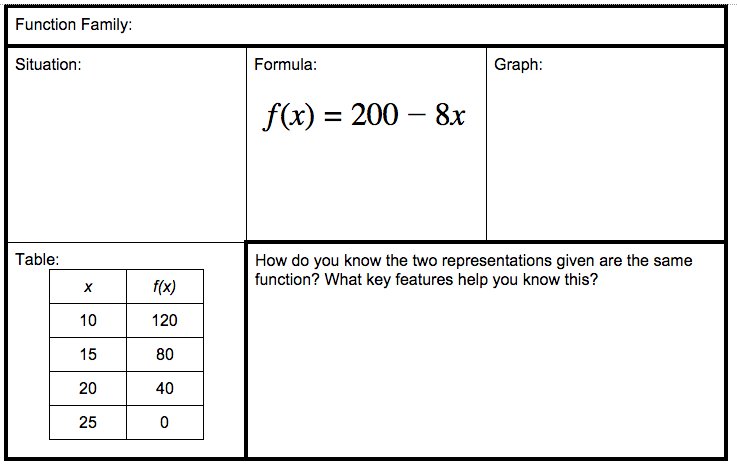
Evidence of Understanding
- analyze equivalent representations of the same function
-
identify characteristics of a function across different representations, especially end behavior, symmetry, critical points (including distinguishing mins and maxs as relative or absolute), zeros, rate of change, and increasing/decreasing/positive/negative intervals
- Example: recognize increasing output values in the table correspond to an increasing interval on the graph, etc.
- relate features of a parabola to features of its quadratic equation
- relate the zeros of factorable polynomial functions to its equation (Note: this supports the Remainder Theorem in the Unit 4)
- articulate and compare the advantages of different representations, including graphs, tables of values, equations, written descriptions, symbolic notations, etc.
-
identify characteristics of a function across different representations, especially end behavior, symmetry, critical points (including distinguishing mins and maxs as relative or absolute), zeros, rate of change, and increasing/decreasing/positive/negative intervals
- create equivalent representations for a polynomial, exponential, piecewise or step function
- identify missing characteristics for one representation using features from a different representation of the same function
-
create an equivalent representation given a graph, table of values, or situation
- justify why both representations are equivalent
- correctly label all important parts of each representation and describe how these labels translate across equivalent representations
- use rates of change to generate equivalent representations
- compare properties of two functions each represented in a different way
- use general characteristics of function families to distinguish and compare the functions
-
describe characteristics that are similar and different
- Example: identify which function has a larger maximum, more zeros, etc.
Develop conceptual understanding:
end behavior, symmetry, critical point, zero, rate of change, polynomial function
Supporting terms to communicate:
domain, range, interval, intercepts, roots, relative/absolute min and max, linear, quadratic, exponential, logarithmic, square root, cube root, polynomial, rational, periodic