Probability

7 Components
Initial Task
Flora, Freddy, and the Future
Big Ideas
Big Idea 1 Big Idea 2
Formative Assessment Lesson
Medical Testing
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 5 Find aligned Regents questions with Quiz Banker End of Unit Assessment Teacher Resources ALG II Unit 5
A2 U5: Probability
Browse Components
Initial Task
Flora, Freddy, and the Future
Big Ideas
Big Idea 1 Big Idea 2
Formative Assessment Lesson
Medical Testing
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 5 Find aligned Regents questions with Quiz Banker End of Unit Assessment Teacher Resources ALG II Unit 5
Big Idea:

Big Idea 2

Probability calculations can be applied to solve problems and make decisions.

1 week
See Standards
Common Core: Supporting Standards:
N-Q.A.2

Reason quantitatively and use units to solve problems. Define appropriate quantities for the purpose of descriptive modeling.

S-CP.B.6

Use the rules of probability to compute probabilities of compound events in a uniform probability model. Find the conditional probability of A given B as the fraction of B's outcomes that also belong to A, and interpret the answer in terms of the model.

S-CP.B.7

Use the rules of probability to compute probabilities of compound events in a uniform probability model. Apply the Addition Rule, P(A or B) = P(A) + P(B) - P(A and B), and interpret the answer in terms of the model.

Common Core: Additional Standards:
S-CP.A.4

Understand independence and conditional probability and use them to interpret data. Construct and interpret two-way frequency tables of data when two categories are associated with each object being classified. Use the two-way table as a sample space to decide if events are independent and to approximate conditional probabilities. For example, collect data from a random sample of students in your school on their favorite subject among math, science, and English. Estimate the probability that a randomly selected student from your school will favor science given that the student is in tenth grade. Do the same for other subjects and compare the results.

S-CP.A.5

Understand independence and conditional probability and use them to interpret data. Recognize and explain the concepts of conditional probability and independence in everyday language and everyday situations. For example, compare the chance of having lung cancer if you are a smoker with the chance of being a smoker if you have lung cancer.

Common Core: Plus Standards:
S-MD.B.5(+)

Use probability to evaluate outcomes of decisions. Weigh the possible outcomes of a decision by assigning probabilities to payoff values and finding expected values.

S-MD.B.6(+)

Use probability to evaluate outcomes of decisions. Use probabilities to make fair decisions (e.g., drawing by lots, using a random number generator).

S-MD.A.2(+)

Calculate expected values and use them to solve problems. Calculate the expected value of a random variable; interpret it as the mean of the probability distribution.

S-MD.A.3(+)

Calculate expected values and use them to solve problems. Develop a probability distribution for a random variable defined for a sample space in which theoretical probabilities can be calculated; find the expected value. For example, find the theoretical probability distribution for the number of correct answers obtained by guessing on all five questions of a multiple-choice test where each question has four choices, and find the expected grade under various grading schemes.

S-MD.A.4(+)

Calculate expected values and use them to solve problems. Develop a probability distribution for a random variable defined for a sample space in which probabilities are assigned empirically; find the expected value. For example, find a current data distribution on the number of TV sets per household in the United States, and calculate the expected number of sets per household. How many TV sets would you expect to find in 100 randomly selected households? 

Evidence of Understanding​​

  • compare probabilities of different events occurring and describe their effect on each other
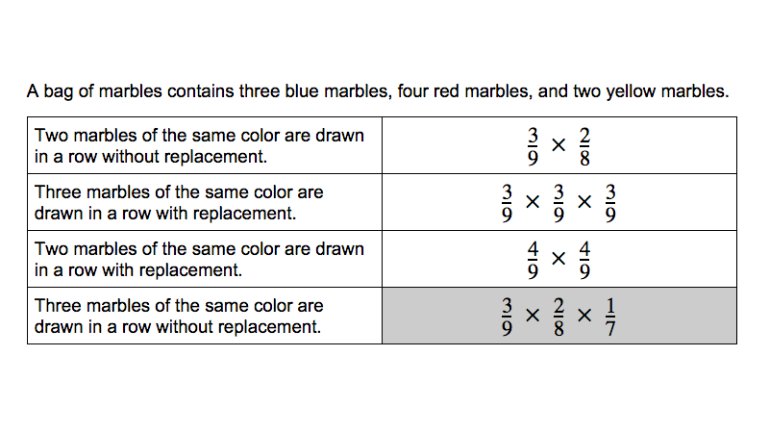
    • categorize and justify if/when events are mutually exclusive (when the probability of the events occurring simultaneously is 0), dependent (including both unions and intersections of sample spaces), or independent
      • analyze and relate the multiplication rule and addition rule to the sample space and relationship between events
    • show that events are independent if and only if the product of their probabilities is equal to the joint probability of the events occurring
    • articulate how one event affects a subsequent event (conditional probability) when events are independent or dependent
    • explain how the correct probability formula describes a desired operation/analysis on the sample space
  • use probability to predict and evaluate outcomes and expected values of decisions
    • determine which probability formula should be used to predict outcomes for a given context
    • apply probability rules to a given context and interpret the meaning of the outcomes within that context
      • (+) weigh the possible outcomes of a decision by assigning probabilities to payoff values and finding expected values.
    • calculate the expected payoff for a game of chance
      • Example: find the expected winnings from a state lottery ticket or a game at a fast-food restaurant
    • use probability and outcome values to justify the fairness of a particular system (and if necessary can make recommendations to make the system more fair)
    • evaluate and compare strategies using expected values
      • Example: compare a high-deductible versus a low-deductible automobile insurance policy using various, but reasonable, chances of having a minor or a major accident
    • explain whether a model is consistent with results from a data-generating process
      • Example: a model says a spinning coin falls heads up with probability 0.5. Would a result of 5 tails in a row cause you to question the model?

Develop conceptual understanding:

mutually exclusive, independent, dependent, multiplication rule, addition rule, conditional probability, fairness, expected value

Supporting terms to communicate:

if and only if, sample space, frequency, event space, complimentary, expected value, possible outcomes, simultaneous, union, intersection, permutation, combination, disjoint, compound
Core Resource

A core resource supports multiple days of instruction. For this Big idea, a partial Core Resource is provided as a starting place for teachers.

Instructional Routine: Contemplate then Calculate
These tasks are embedded within the instructional routine called Contemplate then Calculate.