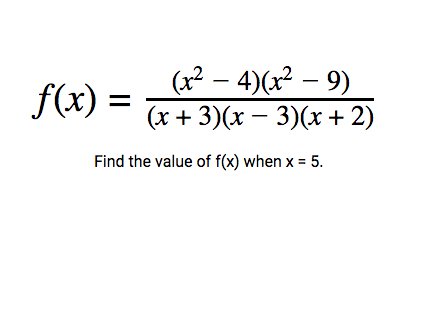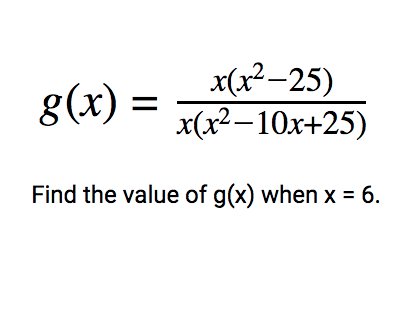Evidence of Understanding
- describe connections between a rational equation and the features of its graph
- describe the general shape and patterns for the graph of a rational function including domain, range, end behavior, and asymptotes
- use the equation to justify vertical asymptotes on the graph (limits on the domain)
- possible extension: use the degree of the numerator and denominator of the rational function to predict oblique asymptotes and use long division to determine their equations
- use the structure of an equation to identify ways to rewrite it and determine asymptotes (mainly via factoring but possibly also applying properties of polynomials)
- solve rational functions in the context of a situation
- build equations that model the relationship between two variables in a rational function
- connect equivalent mathematical representations (an equation to a situation, etc.) and justify the connection
- use multiple mathematical representations (equation, table, graph) to uncover solutions to a problem or answer questions for a given situation
- rewrite rational expressions in different forms using long division when necessary
- Example: (x+4)/(x+3) = (x+3) +1/(x+3) = 1 + 1/(x+3)
- Example: (x3 + 5x2 + 3x - 2)/(x + 1) = x2 + 6x + 9 + 7/(x + 1)
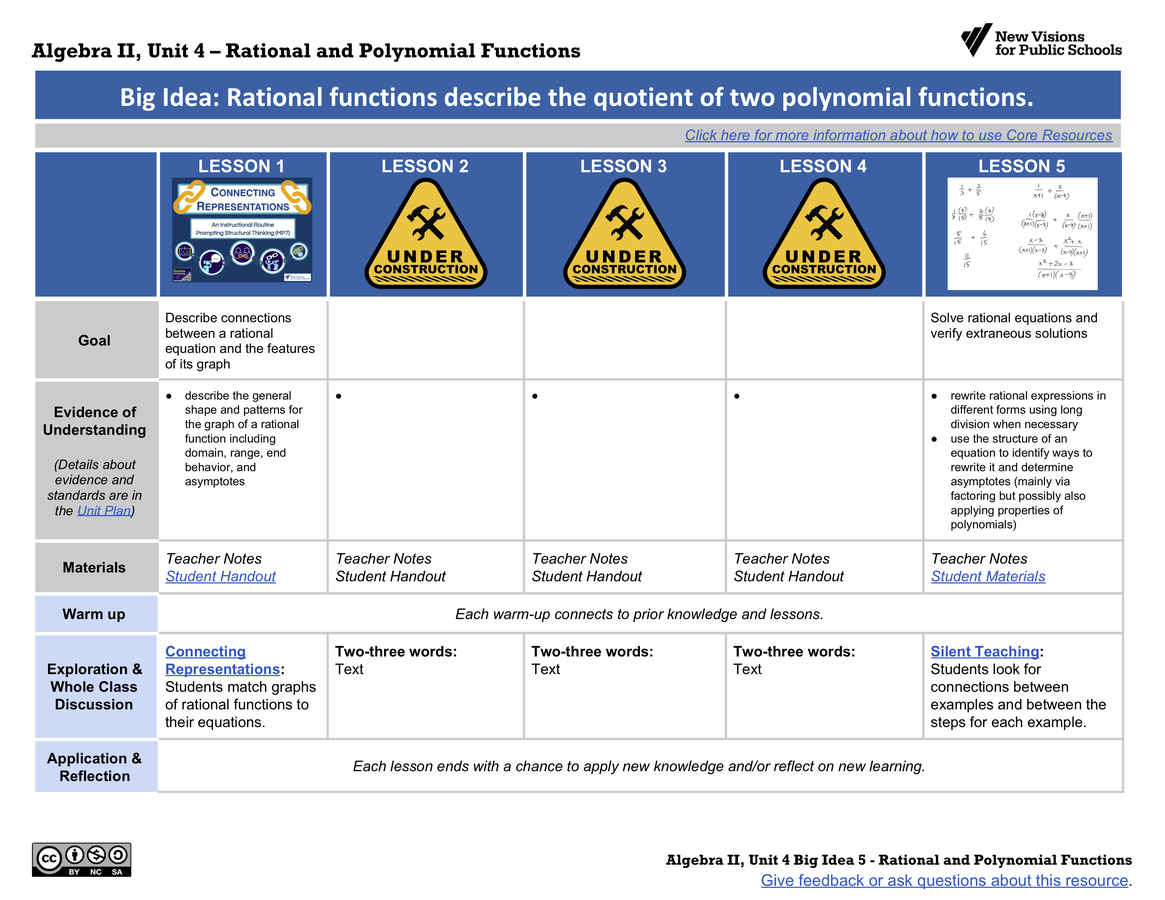
- solve rational equations and verify extraneous solutions
Develop conceptual understanding:
rational function, asymptote, extraneous solution, (+)oblique asymptote
Supporting terms to communicate:
domain, range, end behavior, degree, polynomial, roots, zeros, variable, term, binomial factor