Probability

7 Components
Initial Task
Flora, Freddy, and the Future
Big Ideas
Big Idea 1 Big Idea 2
Formative Assessment Lesson
Medical Testing
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 5 Find aligned Regents questions with Quiz Banker End of Unit Assessment Teacher Resources ALG II Unit 5
A2 U5: Probability
Unit Outline
Initial Task
Flora, Freddy, and the Future
Big Ideas
Big Idea 1 Big Idea 2
Formative Assessment Lesson
Medical Testing
Re-engagement
End of Unit Assessments
End of Unit Assessment for Unit 5 Find aligned Regents questions with Quiz Banker End of Unit Assessment Teacher Resources ALG II Unit 5
None
A2 U5:

Probability

How likely are random events to occur?
3 weeks
See Standards
Common Core: Supporting Standards:
N-Q.A.2

Reason quantitatively and use units to solve problems. Define appropriate quantities for the purpose of descriptive modeling.

S-CP.B.7

Use the rules of probability to compute probabilities of compound events in a uniform probability model. Apply the Addition Rule, P(A or B) = P(A) + P(B) - P(A and B), and interpret the answer in terms of the model.

S-CP.B.6

Use the rules of probability to compute probabilities of compound events in a uniform probability model. Find the conditional probability of A given B as the fraction of B's outcomes that also belong to A, and interpret the answer in terms of the model.

Common Core: Additional Standards:
S-CP.A.1

Understand independence and conditional probability and use them to interpret data. Describe events as subsets of a sample space (the set of outcomes) using characteristics (or categories) of the outcomes, or as unions, intersections, or complements of other events ("or," "and," "not").

S-CP.A.2

Understand independence and conditional probability and use them to interpret data. Understand that two events A and B are independent if the probability of A and B occurring together is the product of their probabilities, and use this characterization to determine if they are independent.

S-CP.A.3

Understand independence and conditional probability and use them to interpret data. Understand the conditional probability of A given B as P(A and B)/P(B), and interpret independence of A and B as saying that the conditional probability of A given B is the same as the probability of A, and the conditional probability of B given A is the same as the probability of B.

S-CP.A.4

Understand independence and conditional probability and use them to interpret data. Construct and interpret two-way frequency tables of data when two categories are associated with each object being classified. Use the two-way table as a sample space to decide if events are independent and to approximate conditional probabilities. For example, collect data from a random sample of students in your school on their favorite subject among math, science, and English. Estimate the probability that a randomly selected student from your school will favor science given that the student is in tenth grade. Do the same for other subjects and compare the results.

S-CP.A.5

Understand independence and conditional probability and use them to interpret data. Recognize and explain the concepts of conditional probability and independence in everyday language and everyday situations. For example, compare the chance of having lung cancer if you are a smoker with the chance of being a smoker if you have lung cancer.

Common Core: Plus Standards:
S-MD.A.2(+)

Calculate expected values and use them to solve problems. Calculate the expected value of a random variable; interpret it as the mean of the probability distribution.

S-MD.A.3(+)

Calculate expected values and use them to solve problems. Develop a probability distribution for a random variable defined for a sample space in which theoretical probabilities can be calculated; find the expected value. For example, find the theoretical probability distribution for the number of correct answers obtained by guessing on all five questions of a multiple-choice test where each question has four choices, and find the expected grade under various grading schemes.

S-MD.A.4(+)

Calculate expected values and use them to solve problems. Develop a probability distribution for a random variable defined for a sample space in which probabilities are assigned empirically; find the expected value. For example, find a current data distribution on the number of TV sets per household in the United States, and calculate the expected number of sets per household. How many TV sets would you expect to find in 100 randomly selected households? 

S-MD.B.5(+)

Use probability to evaluate outcomes of decisions. Weigh the possible outcomes of a decision by assigning probabilities to payoff values and finding expected values.

S-MD.B.6(+)

Use probability to evaluate outcomes of decisions. Use probabilities to make fair decisions (e.g., drawing by lots, using a random number generator).

S-CP.B.8(+)

Use the rules of probability to compute probabilities of compound events in a uniform probability model. Apply the Addition Rule, P(A or B) = P(A) + P(B) - P(A and B), and interpret the answer in terms of the model.

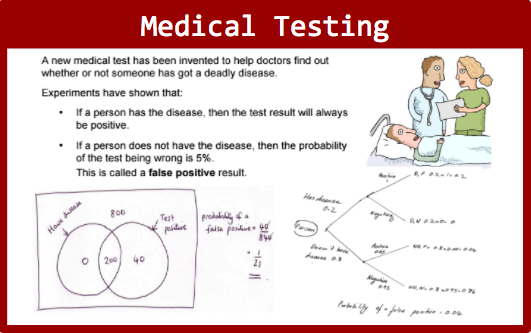
Although students have intuitive notions of how probability works, often their naive mathematical understandings miss critical nuances of probability in action. In this unit, the objective is to confront students with their misconceptions of how probability works and then extend their understanding of probability. This unit builds a foundation for working with probabilities. Basic rules of probability are discussed, including conditional probabilities. Extra care should be taken to develop the conceptual understanding of probability, rather than just memorizing a relationship. Instruction through the use of simulations will help build the necessary conceptual foundation for the successful study of probability. The idea of independence is important and often misunderstood. Being able to explain probability in everyday language as well as interpret everyday language into probability statements is a critical skill for mastering these standards. Students should be exposed to multiple representations of information and asked to calculate probabilities from them. Two-way tables, Venn diagrams, and probability trees should be common in this curriculum.

Essential Questions:

  • How can probabilities be used to analyze and make fair decisions?
  • How likely are random events to occur?
Printable Unit Plan
  • Algebra II Unit Plan for Unit 5
    Resource:
    Algebra II Unit Plan for Unit 5

    This is a printable version of the entire unit plan. You can make a copy of it and edit it or download and print it as needed.

    Preview ResourceAdd a Copy of Resource to my Google Drive
    Type
    Algebra II: Probability
    File
    Google Doc
    Tags
    printable

Unit Outline

7 Components

Every unit begins with an Initial Task and ends with a Balanced Assessment, both focusing on core mathematics of the unit. The core mathematics is developed through a series of resources around Big Ideas; as you move through the unit, keep students focused on how these ideas are connected and how they address mathematical problem solving. Before attempting the Balanced Assessment, students have an opportunity to synthesize their knowledge through a multi-day Formative Assessment Lesson and teacher-designed re-engagement lessons.

Initial Task See 1 itemHide 1 item

Big Ideas See 2 itemsHide 2 items

Formative Assessment Lesson See 1 itemHide 1 item

Re-engagement See 0 itemsHide 0 items

End of Unit Assessments See 3 itemsHide 3 items